If you intersect 3 golden rectangles, their corners can form a regular dodecahedron! But what if you extend the tiles to S shapes and then impossibly tile those?
#Tiling #MathArt #Geometry #MathsArt #Dodecahedron
n-gons on Nostr: #TilingTuesday Dodecahedral tangle. If you intersect 3 golden rectangles, their ...
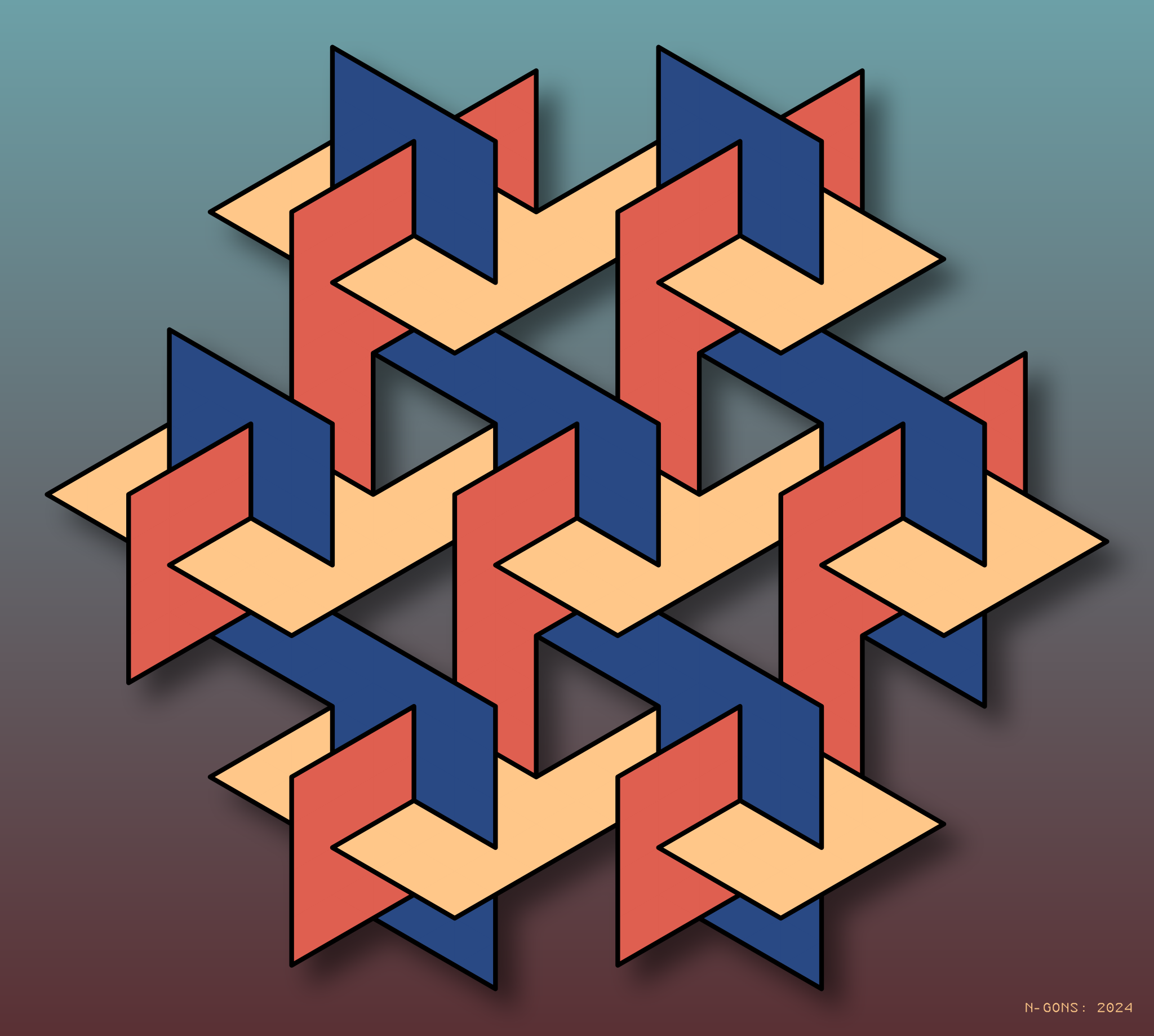
#TilingTuesday Dodecahedral tangle.
If you intersect 3 golden rectangles, their corners can form a regular dodecahedron! But what if you extend the tiles to S shapes and then impossibly tile those?
#Tiling #MathArt #Geometry #MathsArt #Dodecahedron
If you intersect 3 golden rectangles, their corners can form a regular dodecahedron! But what if you extend the tiles to S shapes and then impossibly tile those?
#Tiling #MathArt #Geometry #MathsArt #Dodecahedron