As the 50th captain, say Bob, falls into the black hole, he sees 49 starships in front of him and 49 behind him. This is true at all times: *before* he crosses the horizon, *when* he crosses it, and *after* he crosses it.
Captain Bob never sees any starship cross the horizon until he crosses it. At the moment he crosses the horizon, he sees all 49 starships ahead of him also crossing it — but not the 49 behind him.
However, when any of the starships behind him crosses the horizon, the captain of *that* starship will see Bob also crossing the horizon!
Captain Bob never sees any starship hit the singularity — not even the 49 starships in front of him. The singularity is always in his future, until he hits it.
Thus, as Captain Bob falls into the black hole, he sees his partner, Captain Alice, in front of him for the rest of his short life. But how much is she redshifted?
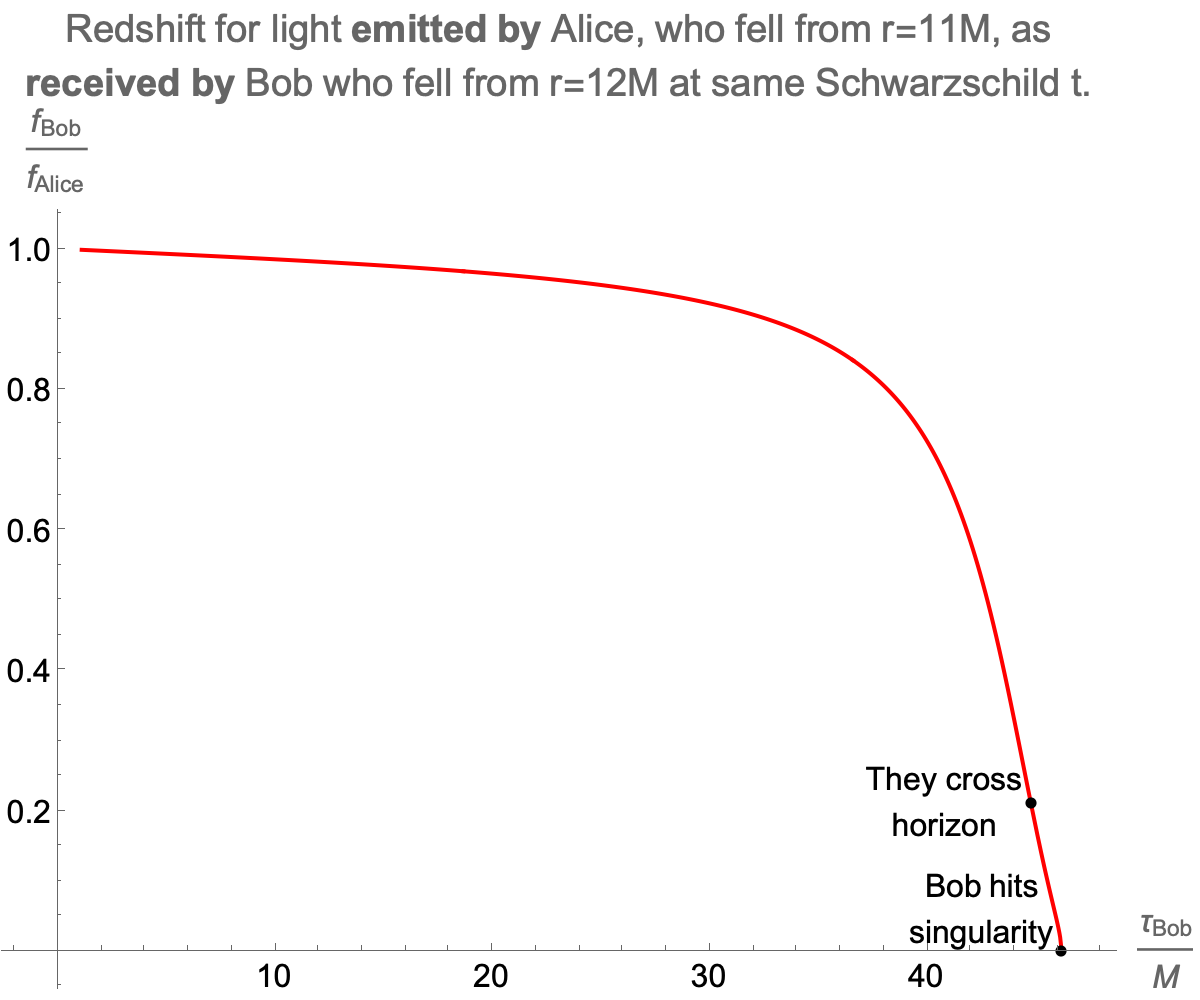
Greg Egan made some assumptions and graphed the result. This shows frequency of light Bob sees divided by the frequency of light Alice emitted, as a function of time as ticked off by Bob’s watch.
Thus, “1” means no redshift at all, and smaller numbers means Alice looks *more* redshifted to Bob!
So: Alice as seen by Bob becomes more and more redshifted as time goes by. She becomes infinitely redshifted at the exact instant Bob hits the singularity!
More details here:
https://johncarlosbaez.wordpress.com/2024/11/30/black-hole-puzzle/